Are Fibonacci Numbers and Golden Ratio Related to Nature and Arts?
For thousands of years we have tried to solve the world we see and to reproduce it using mathematical formulas, or to shape it with the help of math. We already know that the ancient Egyptian architecture was constructed with extreme precision and we know that physicians have proven reality through numbers.
There is a mathematical sequence that has inspired humanity for centuries and which has been a hallmark to define beauty: the Fibonacci numbers. These numbers form a sequence where the next number of the progression is the sum of the two previous, starting from 1 and 1. This means that if you add 1 + 1 = 2, then 2 + 1 = 3, 3 + 2 = 5 and so on. At the end, you’ll end up with this sequence:
1; 1; 2; 3; 5; 8; 13; 21… 55… 1597, and so on.
Something that intrigued both artists and scientist is that if you divide a number of the series by the previous one in the line, the bigger the better, you have a number close to the golden ratio, that is the irrational number
? = 1/2 (1+5)= 1,6180339….
In other words: two quantities are in golden ratio if their ratio is the same as the ratio of their sum to the larger of the two quantities. The ratio that can fulfill this statement is the infinite number above.
The Fibonacci sequence was described around 1202 by the Italian mathematician Leonardo of Pisa, better known as Fibonacci, but it’s been already known in India and it’s been used in poetry and math. Although we don’t know when the golden ratio was first used, we know for certain that we use its geometrical representation since at least 300 BC, when Euclid first mentioned it. Over time, we started to recognize the ratio and the numerical sequence everywhere, from geometry statements to mathematical relations, from arts to architecture, from biology to music. Sometimes the evidence is clear and obvious, sometimes it is an assumption.
the fibonacci number and the golden section in nature...
You may have heard that the golden means can be found everywhere in nature, usually supported by the Fibonacci sequence. There’s a small truth to this statement, but it’s actually wrong! We can find the Fibonacci numbers in a lot of nature patterns, like in the stripes of a zebra or in the flowering of an artichoke, but that is just an evolutionary ploy. Since irregular shapes can often provide a better chance to survive (if your leaves are positioned irregularly, they will get more sun, how convenient it is!), nature found the laziest way to overcome the problem! This can happen in different ways: some of the shapes are regular, some of them are irregular. The easiest way to cover a surface is by using hexagons, as in the eyes of dragonflies, while growing a resistant shell to cover your body needs less energy if it’s spiral shaped!
Those spirals are actually the ones that are more mistaken and seen as made with the golden ratio. They may seem like the golden spiral, but they are just an approximation of the ancient ratio.
-

The zebra stripes follow the Fibonacci sequence. Photo by Ron Dauphin -

The nautilus shell isn’t actually related to the golden ratio. Photo by Shaun Low
IN ARTS...
Proportions can be found in architecture or in arts, as in poetry or music. For example Polykleitos used a ratio developed to create what he thought was beauty, the Canon of Polykleitos. Poetry usually has canonic metric as praxis just like most music genres. Some people think that Phidias’ Parthenon has the proportion calculated with the golden section, but that seems to be another myth. That’s not a big mistake, since ancient Greeks were lovers of proportion, and it’s not unlikely that the architect did use some math to build it.
During Renaissance, artists started exploring the golden mean and other ratios, as after humanism the interest for beauty in the world increased. Pacioli’s Divina Proportione, the first book that explains the golden section, has been illustrated by Leonardo da Vinci. He called this ratio as sectio aurea for the first time, which means – of course – golden section!
From Piet Mondrian to Jacques Villon, from le Corbusier to Mario Botta; countless artists have used the Fibonacci sequence in their art. These artworks provoked a growing interest for mathematical principles. The importance of math in art increased, becoming a must for some artists such as Mario Merz, whose works are mostly related to the Fibonacci numbers.

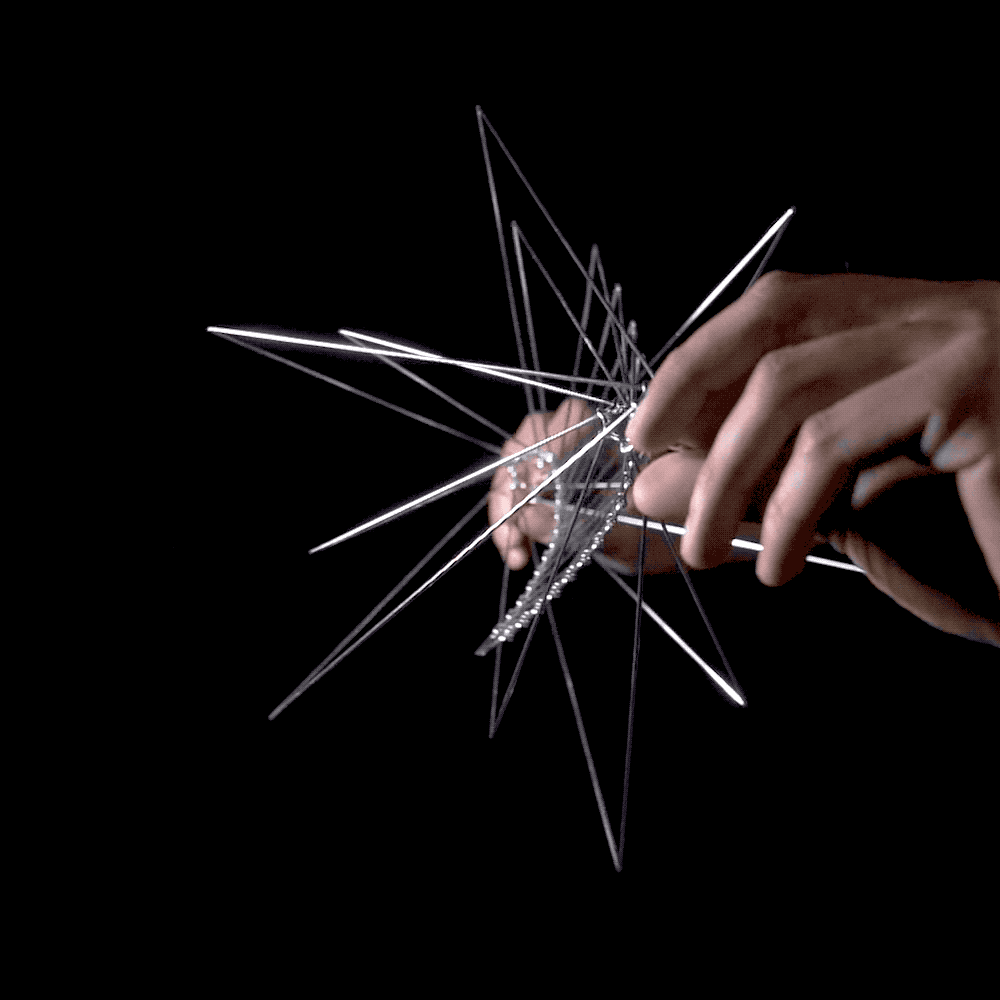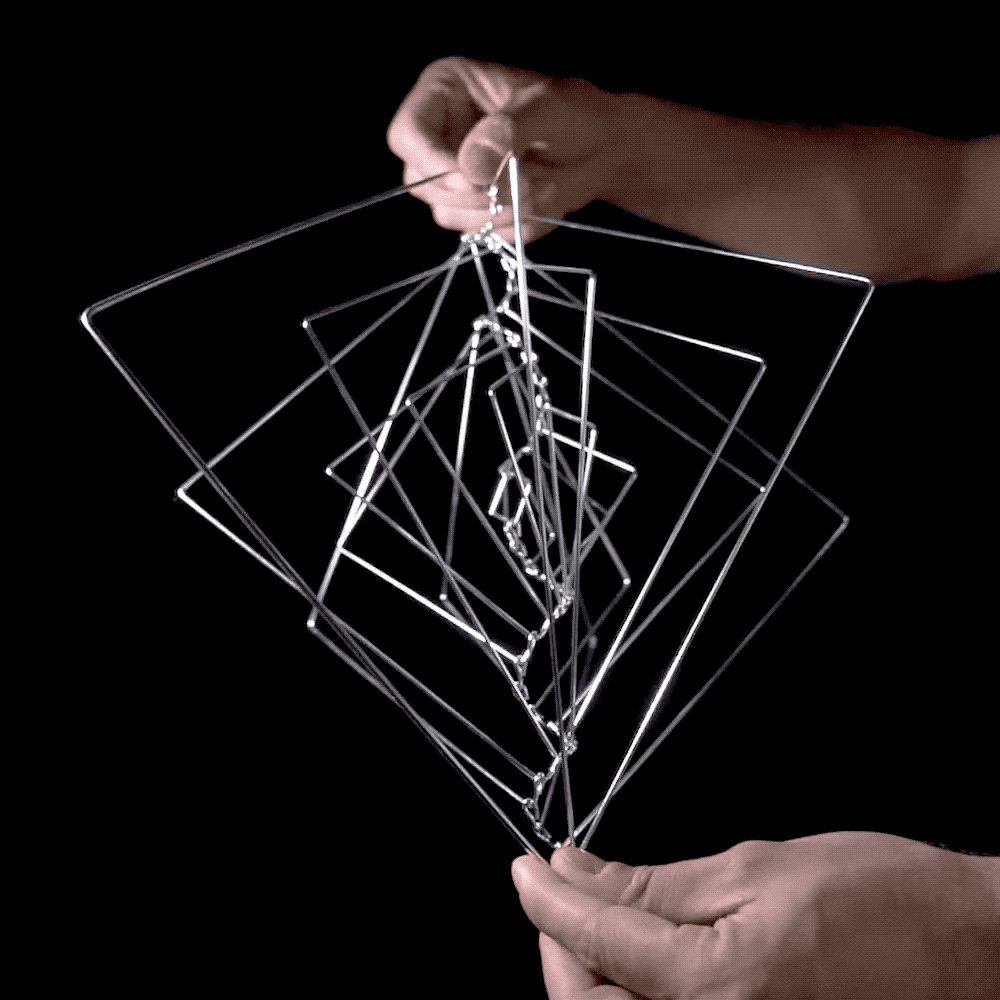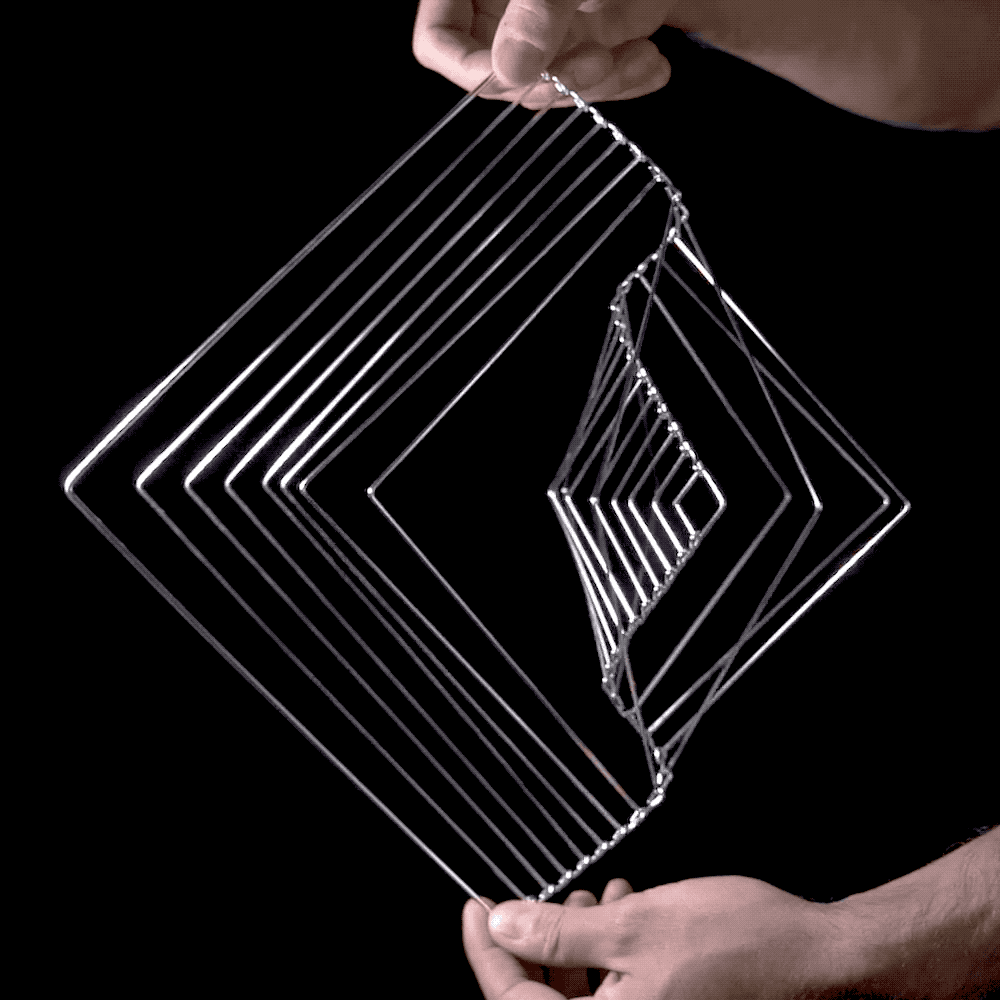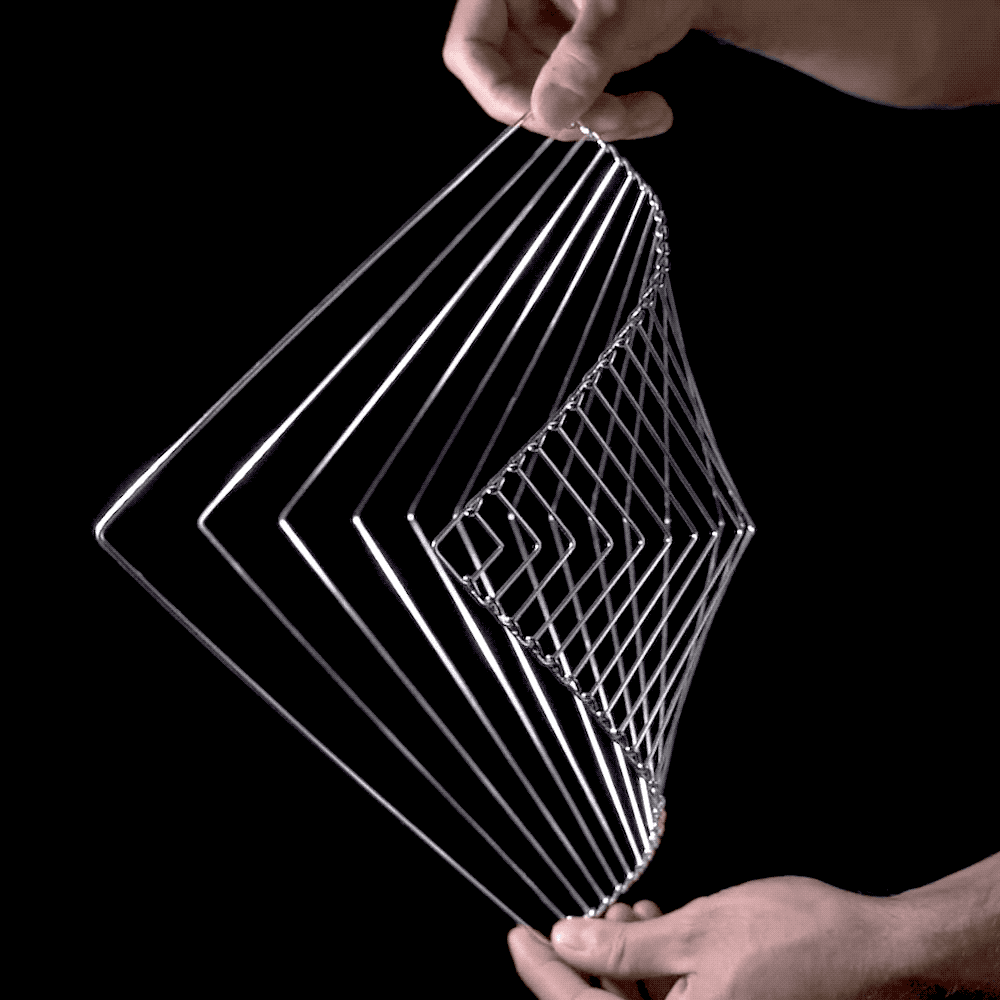
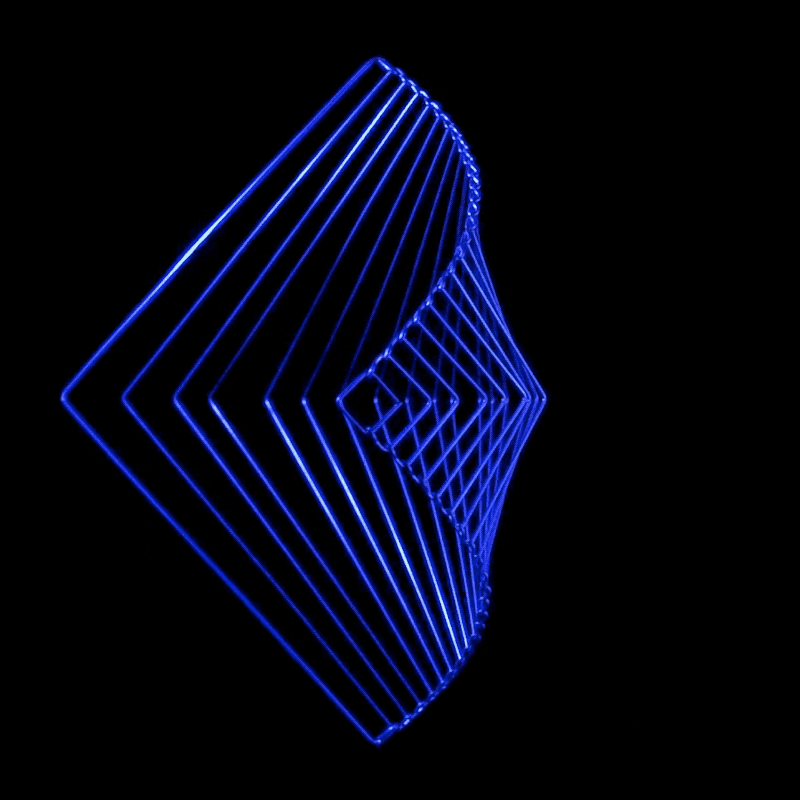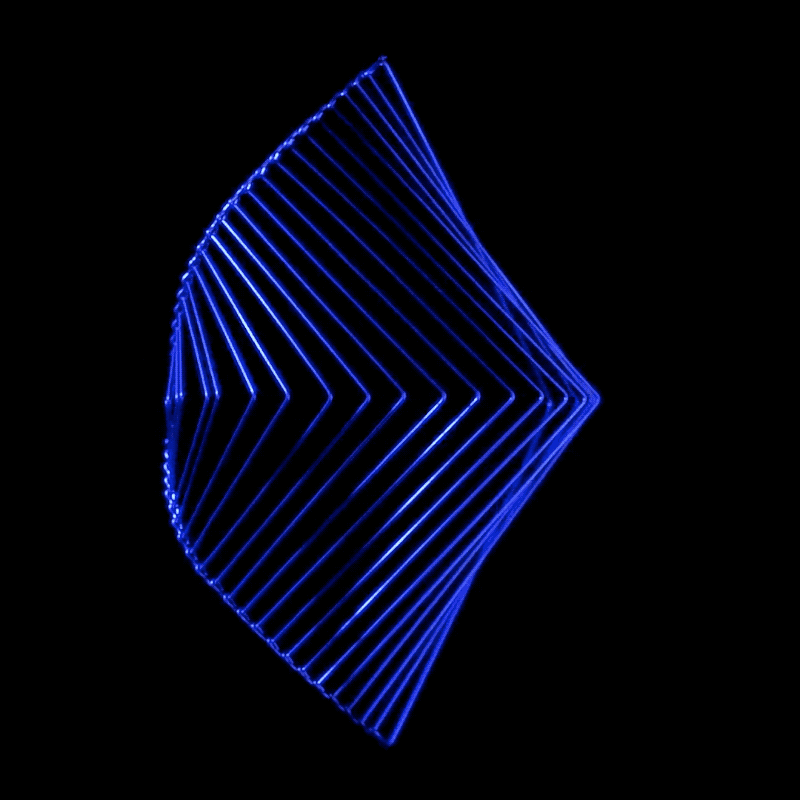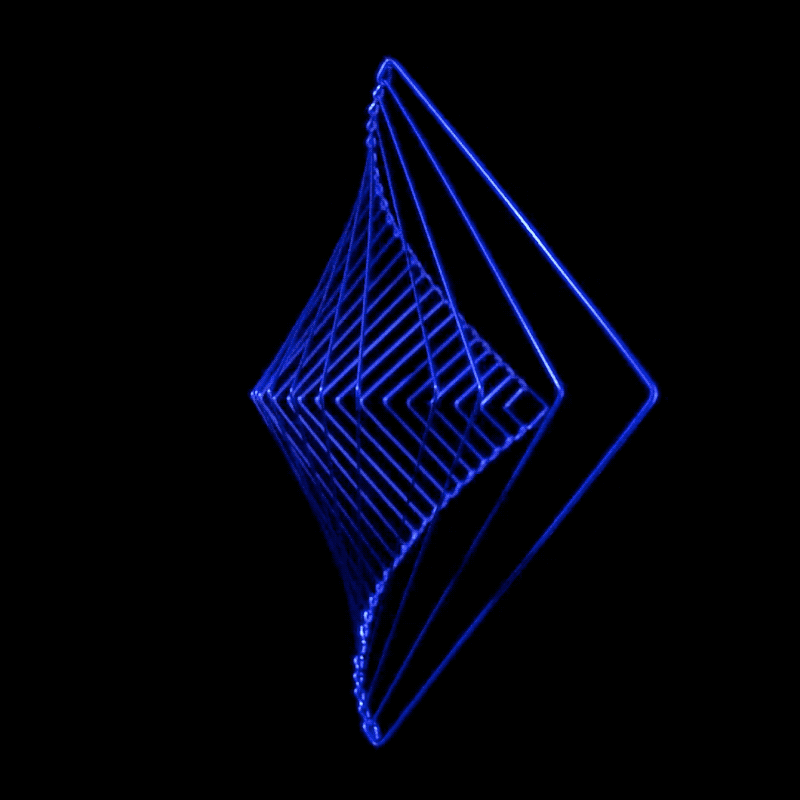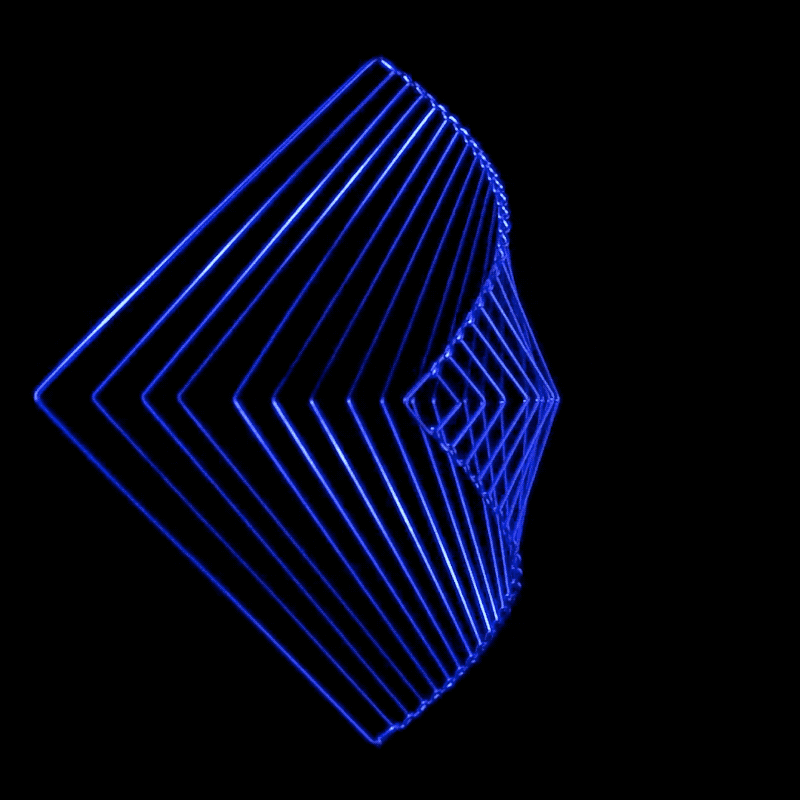
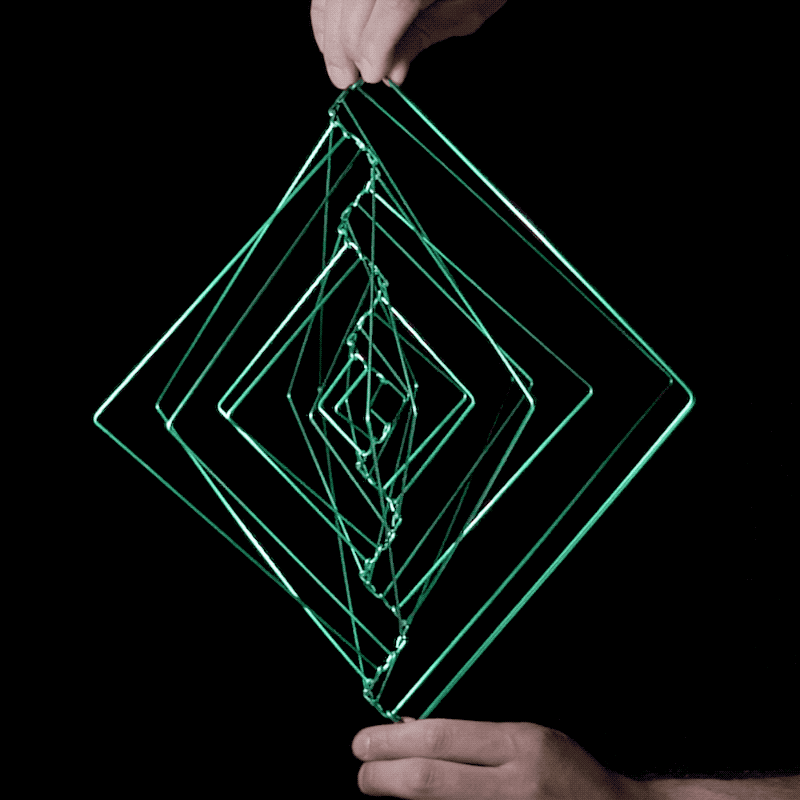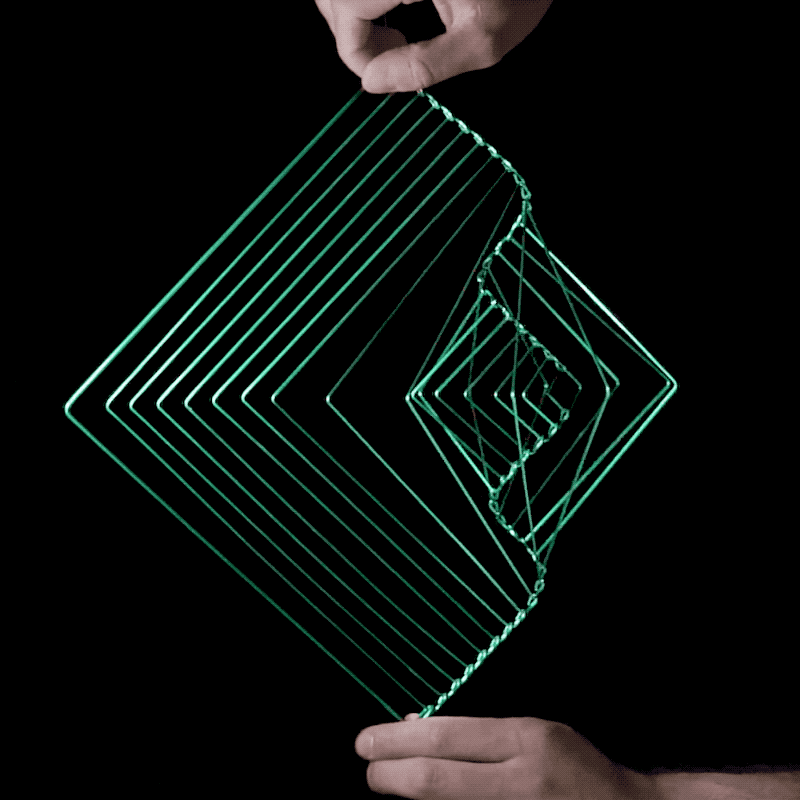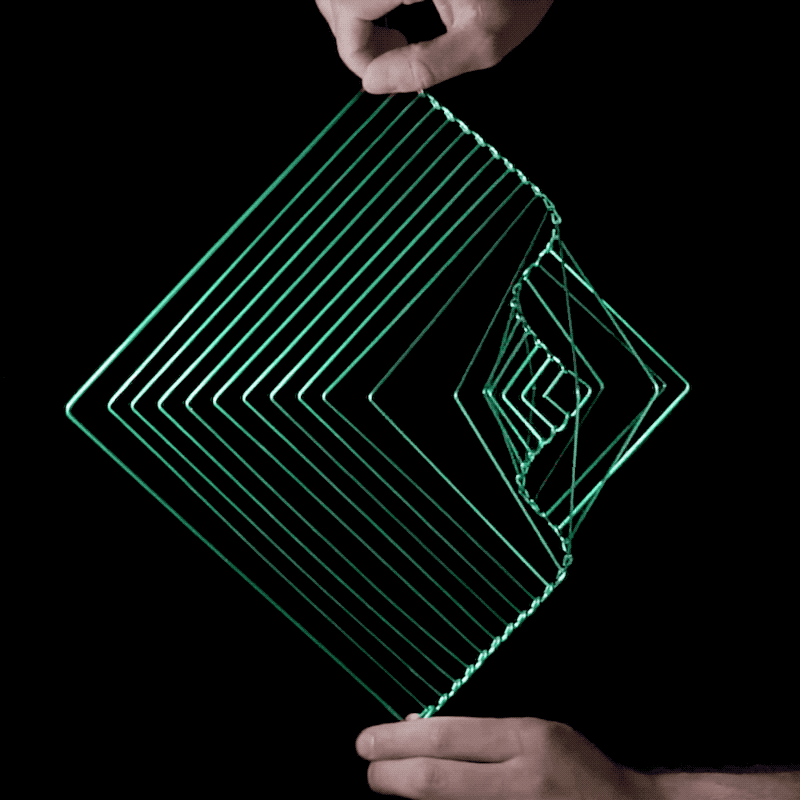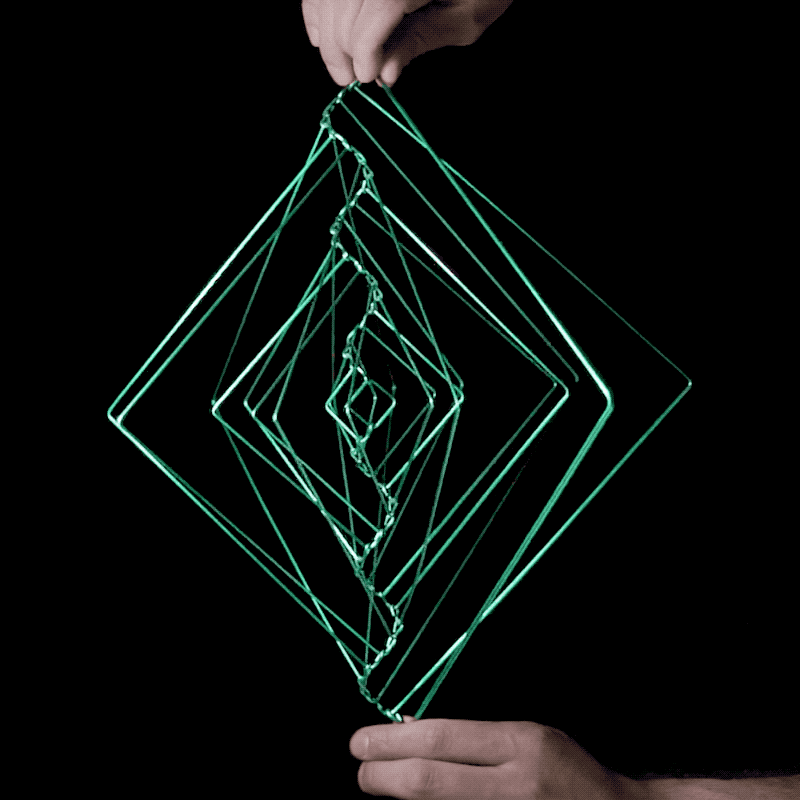
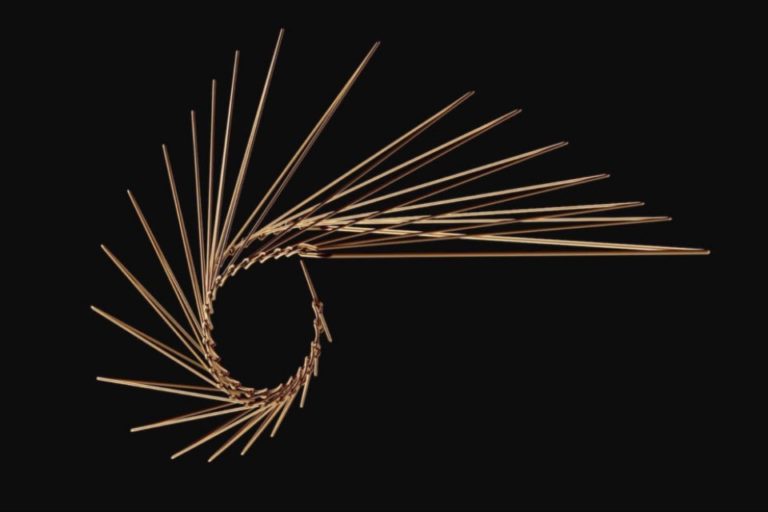
Another artist that is fascinated by the sequence is Ivan Black, a kinetic sculptor who uses the series to model his sculptures into a hypnotic, multi-shaped wave. Most of his works don’t seem to have the series in it, but when you spin them, you’ll see golden spirals form in front of your eyes!
In music, we can also find some other examples of using the golden ratio or the Fibonacci numbers. Béla Bartók probably used the sequence in his Music for Strings, Percussion and Celesta and other compositions, just as Erik Satie for his Trois sonneries de la Rose+Croix. Even Claude Debussy, the composer of Images and the famous Clair de Lune had divided the sections of his Nocturne n°1, also called “Nuages” by the Fibonacci Numbers.

... EVERYWHERE
The golden section and the Fibonacci numbers have impressed great minds and gave us some of the most beautiful artworks we can enjoy today. It doesn’t matter if mostly we have approximations or adaptations of this mathematical properties, but since even nature prefers imperfection to solve his problems, the results of these researches are often astonishing. So, be curious about innovation and mathematics so you can see a different beauty behind things. The universe spins in spirals while Ivan Black’s little artworks spin inside a little room, and spinning we go further and further with great ideas inspired by the surrounds!